Euclid’s Postulates

Geometry is in everything. The first attempts at understanding this art of nature were made even before recorded history. However, the most notable and influential was the Euclid Geometry. Euclid of Alexandria developed one of the most beautiful and the most interesting treatise of mathematics – Elements. What is written in this book? How did it play a role in the development of Science and mathematics? Let us see!

Suggested Videos

 

Euclid’s Postulates

Any statement that is assumed to be true on the basis of reasoning or discussion is a postulate or axiom.  The postulates stated by Euclid are the foundation of Geometry and are rather simple observations in nature. ‘Euclid’ was a Greek mathematician regarded as the ‘Father of Modern Geometry‘.

He is credited with profound work in the fields of algebra, geometry, science, and philosophy. Euclid introduced the fundamentals of geometry in his book called “Elements”. There are 23 definitions or Postulates in Book 1 of Elements (Euclid Geometry). We will see a brief overview of some of them here. Their order is not as in Elements.

Postulate – I

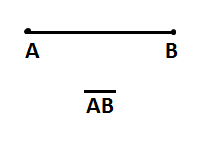
A straight line segment can be formed by joining any two points in space.

In Geometry, a line segment is a part of a line that is bounded by 2 distinct points on either end. It consists of a series of points bounded by the two endpoints. Thus a line segment is measurable as the distance between the two endpoints. A line segment is named after the two endpoints with an overbar on them.

Postulate – II

Any straight line can be extended indefinitely on both sides. Unlike a line segment, a line is not bounded by any endpoint and so can be extended indefinitely in either direction. A line is uniquely defined as passing through two points which are used to name it.

Postulate – III

A circle can be drawn with any centre and any radius. For any line segment, a circle can be drawn with its centre at one endpoint and the radius of the circle as the length of the line segment. Consider a line segment bounded by two points. If one of these points is taken as the centre of a circle and the radius of the circle is taken as equal to the length of the segment, a circle can be drawn with its diameter twice than the length of the line segment.

In the above example, the line segment AO serves as the radius of a circle with centre at point O and a diameter equal to AB where l(AB) =2l(AO).

Postulate – IV

All right angles are congruent or equal to one another. A right angle is an angle measuring 90 degrees. So, irrespective of the length of a right angle or its orientation all right angles are identical in form and coincide exactly when placed one on top of the other.

A right angle

Postulate – V

Two lines are parallel to each other if they intersect the third line and the interior angle between them is 180 degrees.

‘Parallel lines’ are a set of 2 or more lines that never cross or intersect each other at any point in space if they are extended indefinitely. As you can see in the above image, line 1 and line 2 are parallel if and only if the sum of angles ‘a’ and ‘b’ they make with the transversal is 180 degrees.

You can download Introduction to Euclid’s Geometry Cheat Sheet by clicking on the download button below

Solved Examples For You

Question 1: Euclid’s fifth postulate is

1. The whole is greater than the part.
2. A circle may be described with any radius and any centre.
3. All right angles are equal to one another.
4. If a straight line falling on two straight lines makes the interior angles on the same side of it, taken together = less than two right angles (<180⁰), then the two straight lines if produced indefinitely,  meet on that side on which the sum of angles is less than two right angles.

Answer : D)The fifth postulate talks about the condition of two lines being parallel. In the figure below, as you can see that as α + β < 180, the two lines when produced meet the side on which the sum of angles is less than two right angles (shown here by dots).

Question 2: State the five postulates of Euclidean geometry?

Answer: Five common postulates of Euclidean geometry are:

• You can draw a straight-line segment from any given point to others.
• You can extend a straight-line to a finite length.
• It can be described as a circle with any given point as its center and any distance as its radius.
• In it all right angles are congruent.
• Lastly, if the straight-line intersect two other straight-lines, and make the two interior angles on one side of it together less than two right angles, then the other straight line will meet at a point on the side with less than two right angles.

Question 3: What are the uses of Euclidean geometry?

Answer: We use Euclidean geometry to design buildings, predict the location of moving objects, and survey land.

Question 4: How Euclid contribute to geometry?

Answer: From a small set of axioms Euclid deduced the theorems of what is now known as Euclidean geometry. In addition, he also wrote works on conic sections, number theory, mathematical rigor, perspective, and spherical geometry.

Question 5: How does Euclid define a line?

Answer: When Euclid first formalized the geometry, he defined a general line as “breathless length” as a straight line is a line “that lies evenly with the points on itself” in two dimensions.