Increasing and Decreasing Functions

A better understanding of real-life processes is obtained by expressing them in the form of functions of the known variables which control those processes. Their analysis is then done by analyzing their behavior when the variables change. Whether the functions increase or do they decrease? That is what we are going to understand in this topic of increasing and decreasing functions.

Suggested Videos

You could be able to relate this to the rate of change of quantities topic. Indeed, there is a connection between the two. This topic actually enhances the applicability of the rate of change of quantities you learned to obtain.

For example, if we assign a function ‘speed‘ to the speed of a moving train and measure the rate of change in speed with respect to time as 10 meters/second in a time interval of 1 second, we can understand that the function speed behaves like an increasing function in the interval of 1 second from its initial point.

Such analysis is crucial in determining the nature of the speed of the train over different intervals of time irrespective of the values you obtain for the rate of change of speed.

Browse more Topics under Application Of Derivatives

• Rate of Change of Quantities
• Approximations
• Maxima and Minima
• Tangents and Normals

Increasing and Decreasing Functions

Let y = f(x) be a differentiable function (whose derivative exists at all points in the domain) in an interval x = (a,b).

• If for any two points x₁ and x₂ in the interval x such that x₁ < x₂, there holds an inequality f(x₁) ≤ f(x₂); then the function f(x) is called increasing in this interval.
• Similarly, if for any two points x₁ and x₂ in the interval x such that x₁ < x₂, there holds an inequality f(x₁) ≥ f(x₂); then the function f(x) is called decreasing in this interval.

The functions are known as strictly increasing or decreasing functions, given the inequalities are strict: f(x₁) < f(x₂) for strictly increasing and f(x₁) > f(x₂) for strictly decreasing. Look at the possible shapes of various types of increasing and decreasing functions below:

 Monotonic Function

If a function is differentiable on the interval (a,b) and falls in any one of the four categories explained above i.e. Increasing/strictly Increasing, Decreasing/strictly decreasing, then the function is known as a Monotonic function. Note that if \({\frac{df}{dx} = 0}\), the function is constant on that interval.

The First Derivative Test

This test can be used to find the nature of the function i.e. whether it is monotonic or not. It employs the use of the derivative of the function with respect to the independent variable at a point in the interval where the behavior is to be determined. For a function f(x) in the domain (a,b): $$ {{\text{If }}{\frac{df}{dx} \geq 0} \text{ for all x in (a,b), then f(x) is an Increasing Function in (a,b).}} $$ Similarly, $$ {{\text{If }}{\frac{df}{dx} \leq 0} \text{ for all x in (a,b), then f(x) is an Decreasing Function in (a,b).}} $$

For the strictly monotonic nature, strict inequalities must hold i.e.

• \( { \frac{df}{dx} > 0} \) for Strictly Increasing Functions
• \( { \frac{df}{dx} < 0} \) for Strictly Decreasing Functions

Proof of the First Derivative Test

We will prove the test for Increasing Functions and leave the part for Decreasing Functions up to you. The test says:

\( {{\text{If }}{\frac{df}{dx} \leq 0}} \) for all x in (a,b), then f(x) is an Increasing Function in (a,b). Recount the definition of the derivative of a function: $$ {\frac{df}{dx} = _{\Delta{x} \rightarrow{0}} {\frac{f(x + \Delta x) – f(x)}{\Delta x}}} $$

If we assume that Δx > 0, then the derivative i.e. \({\frac{df}{dx} /geq 0}\) would be greater than 0 only if the numerator on the R.H.S. of the definition is greater than 0. It would then imply that  f(x + Δx) ≥ f(x), which means that f(x) is an increasing function! Similarly, other cases can be proved.

Solved Examples for You

Question 1: What is the nature of the curve sin x in (0,π/4) and (π/4,π/2)?

Answer : We will need to calculate the derivative of the function sin x to proceed, $$ {\frac{d(sin x)}{dx} = cos x} $$ Here is the graph of cos x:

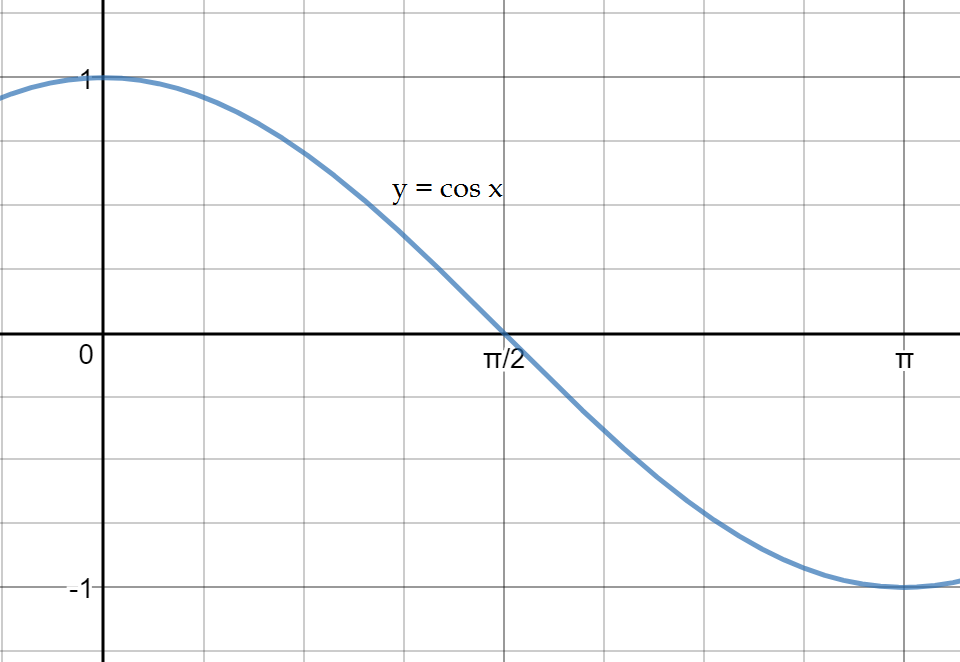
From the graph, one can easily analyse that:

• For x ∈ (0,π/4), cos x > 0 i.e. \( {\frac{d(sin x)}{dx} > 0}\) . Thus, sin x is a Strictly Increasing Function in  (0,π/4).
• For x ∈ (0,π/4), cos x < 0 i.e. \( {\frac{d(sin x)}{dx} < 0}\) . Thus, sin x is a Strictly Decreasing Function in  (π/4,π/2).

This concludes our discussion on this topic of increasing and decreasing functions.

Question 2: What does non-decreasing mean?

Answer: Increasing is when every element is greater than the one prior to it. Non-decreasing refers to when no element is less than the element previous to it. Thus, to sum it up, every element is greater than or equal to the one previous to it.

Question 3: What is the interval of decrease?

Answer: Intervals of increase and decrease refer to the domain of a function where the value of it gets larger or smaller, correspondingly.

Question 4: What is end behaviour?

Answer: The end behaviour of a polynomial function refers to the behaviour of the graph of f(x) as x will approach positive infinity or negative infinity. The degree and the leading coefficient of a polynomial function will determine the end behaviour of the graph.

Question 5: What does the second derivative tell you?

Answer: The second derivative tells us a great deal about the qualitative behaviour of the graph. If the second derivative will be positive at a point then the graph is concave up. If the second derivative is going to be positive at a critical point, then the critical point will be a local minimum. Thus, the second derivative is zero at an inflection point