Radioactivity – Law of Radioactive Decay

In 1896, A.H. Becquerel accidentally discovered radioactivity. He was studying the fluorescence and phosphorescence of compounds irradiated with visible light. This is when he observed something interesting. Let’s learn more about the law of radioactive decay in the section below.

Suggested Videos

 

He illuminated some pieces of Uranium-Potassium-Sulphate with visible light. Next, he wrapped these pieces in black paper and separated it from a photographic plate by a piece of silver. He left it for several hours. When he developed the photographic plate, he found that there was blackening on the plate.

This meant that something was emitted by the compound which penetrated the silver and black paper and hit the plate. Subsequent experiments show that radioactivity is a nuclear phenomenon which occurs when an unstable nucleus undergoes a decay. This is called Radioactive Decay.

Browse more Topics under Nuclei

• Atomic Mass and Composition of Nucleus
• Mass-Energy and Nuclear Binding Energy
• Nuclear Force
• Nuclear Energy – Nuclear Fusion
• Nuclear Energy – Nuclear Fission
• Radioactivity – Types of Radioactive Decay

Radioactive Decay

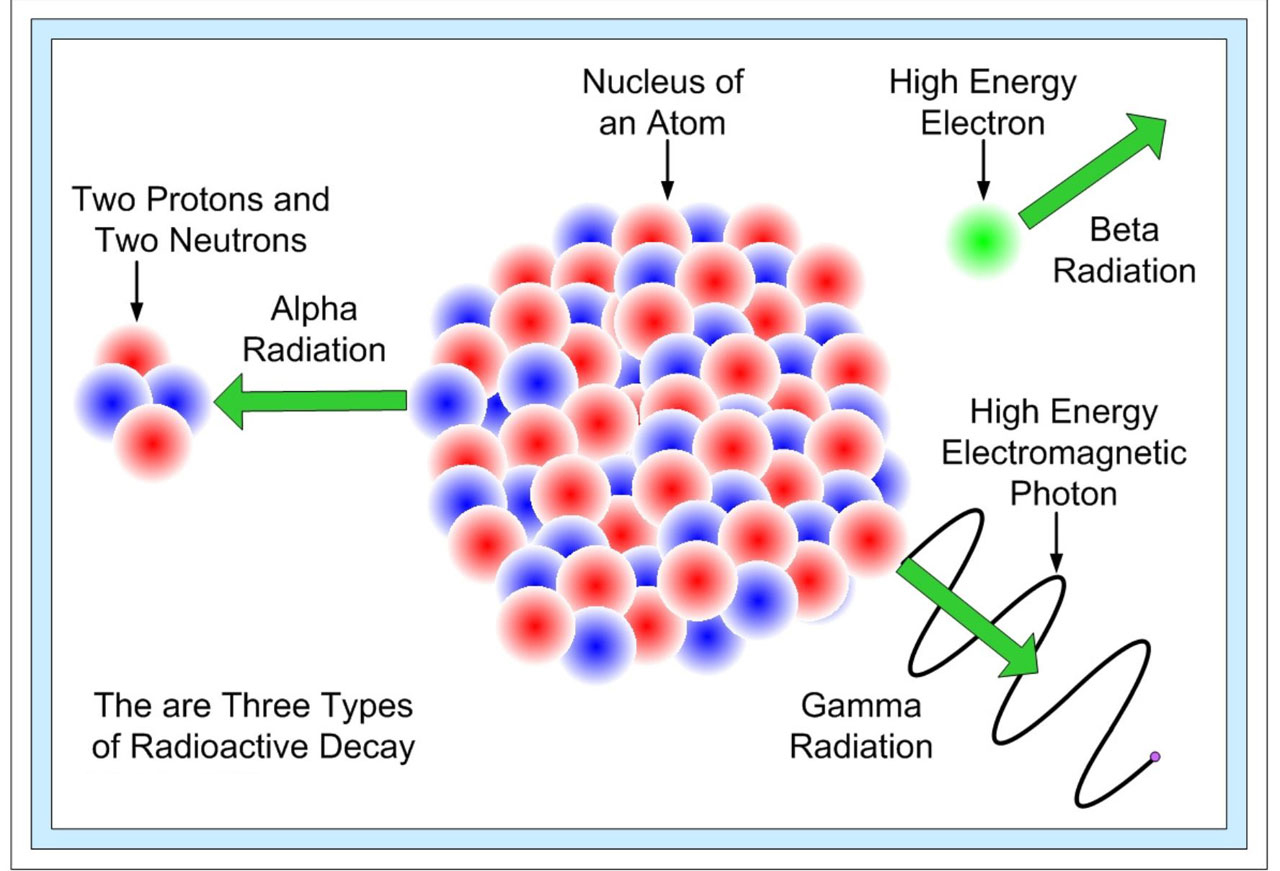
There are three types of radioactive decays in nature:

• α-decay –a helium nucleus (⁴₂He) is emitted
• β-decay – where electrons or positrons (particles with the same mass as electrons, but with a charge exactly opposite to that of an electron) are emitted;
• γ-decay – high energy (hundreds of keV or more) photons are emitted.

Law of Radioactive Decay

When a radioactive material undergoes α, β or γ-decay, the number of nuclei undergoing the decay, per unit time, is proportional to the total number of nuclei in the sample material. So,

If N = total number of nuclei in the sample and ΔN = number of nuclei that undergo decay in time Δt then,

ΔN/ Δt ∝ N

Or, ΔN/ Δt = λN … (1)

where λ = radioactive decay constant or disintegration constant. Now, the change in the number of nuclei in the sample is, dN = – ΔN in time Δt. Hence, the rate of change of N (in the limit Δt → 0) is,

dN/dt = – λN

Or, dN/N = – λ dt

Now, integrating both the sides of the above equation, we get,

^N_N0∫ dN/N = λ ^t_t0∫ dt … (2)

Or, ln N – ln N₀ = – λ (t – t₀) … (3)

Where, N₀ is the number of radioactive nuclei in the sample at some arbitrary time t₀ and N is the number of radioactive nuclei at any subsequent time t. Next, we set t₀ = 0 and rearrange the above equation (3) to get,

ln (N/N₀) = – λt

Or, N(t) = N₀e^{– λt} … (4)

Equation (4) is the Law of Radioactive Decay.

The Decay Rate

In radioactivity calculations, we are more interested in the decay rate R ( = – dN/dt) than in N itself. This rate gives us the number of nuclei decaying per unit time. Even if we don’t know the number of nuclei in the sample, by simply measuring the number of emissions of α, β or γ particles in 10 or 20 seconds, we can calculate the decay rate. Let’s say that we consider a time interval dt and get a decay count ΔN (= –dN). The decay rate is now defined as,

R = – dN/dt

Differentiating equation (4) on both sides, we get,

R = λ N₀ e^−λt

Or, R = R₀e^−λt … (5)

Where, R₀ is the radioactive decay rate at the time t = 0, and R is the rate at any subsequent time t. Equation (5) is the alternative form of the Law of Radioactive Decay. Now we can rewrite equation (1) as follows,

R = λN … (6)

where R and the number of radioactive nuclei that have not yet undergone decay must be evaluated at the same instant.

Half-Life and Mean Life

The total decay rate of a sample is also known as the activity of the sample. The SI unit for measurement of activity is ‘becquerel’ and is defined as,

1 becquerel = 1 Bq = 1 decay per second

An older unit, the curie, is still in common use:

1 curie = 1 Ci = 3.7 × 10¹⁰ Bq (decays per second)

There are two ways to measure the time for which a radionuclide can last.

• Half-life T_1/2 – the time at which both R and N are reduced to half of their initial values
• Mean life τ – the time at which both R and N have been reduced to, e^-1 of their initial values.

Calculating Half-Life

Let’s find the relation between T1/2 and the disintegration constant λ. For this, let’s input the following values in equation (5),

R = (1/2)R₀ and t = T_1/2

So, we get T_1/2 = (ln2)/ λ

Or, T_1/2 = 0.693/ λ … (7)

Calculating Mean life

Next, let’s find the relation between the mean life τ and the disintegration constant λ. For this, let’s consider equation (5),

• The number of nuclei which decay in the time interval: ‘t’ to ‘t + Δt’ is: R(t)Δt = (λN₀e^–λt Δt).
• Each of them has lived for time ‘t’.
• Hence, the total life of all these nuclei is tλN₀e^–λt Δt

Hence, to obtain the mean life, we integrate this expression over all the times from 0 to ∞ and divide by the total number of nuclei at t = 0 (which is N₀).

τ = (λN₀ ⁰_∞∫ te^–λtdt)/N₀

= λ⁰_∞∫ te^–λtdt

On solving this integral, we get

τ = 1/λ

Therefore, we can summarise the observations as follows:

T1/2 = (ln2)/λ = τ ln 2 … (8)

Solved Examples for You

Question: The half-life of ²³⁸₉₂U undergoing α-decay is 4.5 × 10⁹ years. What is the activity of 1g sample of ²³⁸₉₂U ?

Answer: T1/2 = 4.5 x 10⁹ years = 4.5 x 10⁹years x 3.6 x 10⁷ seconds/year = 1.42 x 10¹⁷ seconds

We know that 1 k mol of any isotope contains Avogadro’s number of atoms. Hence, 1g of ²³⁸₉₂U contains, {1/(238 x 10^-3)} x 6.025 x 10²⁶ = 25.3 x 10²⁰ atoms. Therefore, the decay rate R is,

R = λN
= (0.693/T_1/2)N
= (0.693 x 25.3 x 10²⁰) / (1.42 x 10¹⁷)

Therefore, R = 1.23 x 10⁴ Bq